leetcode

README

850. Rectangle Area II

题目

We are given a list of (axis-aligned) rectangles. Each rectangle[i] = [x1, y1, x2, y2] , where (x1, y1) are the coordinates of the bottom-left corner, and (x2, y2) are the coordinates of the top-right corner of the ith rectangle.

Find the total area covered by all rectangles in the plane. Since the answer may be too large, return it modulo 10^9 + 7.

Example 1:

Input: [[0,0,2,2],[1,0,2,3],[1,0,3,1]]
Output: 6
Explanation: As illustrated in the picture.

Example 2:

Input: [[0,0,1000000000,1000000000]]
Output: 49
Explanation: The answer is 10^18 modulo (10^9 + 7), which is (10^9)^2 = (-7)^2 = 49.

Note:

• 1 <= rectangles.length <= 200
• rectanges[i].length = 4
• 0 <= rectangles[i][j] <= 10^9
• The total area covered by all rectangles will never exceed 2^63 - 1 and thus will fit in a 64-bit signed integer.

题目大意

我们给出了一个（轴对齐的）矩形列表 rectangles。 对于 rectangle[i] = [x1, y1, x2, y2]，其中（x1，y1）是矩形 i 左下角的坐标，（x2，y2）是该矩形右上角的坐标。找出平面中所有矩形叠加覆盖后的总面积。由于答案可能太大，请返回它对 10 ^ 9 + 7 取模的结果。

提示：

• 1 <= rectangles.length <= 200
• rectanges[i].length = 4
• 0 <= rectangles[i][j] <= 10^9
• 矩形叠加覆盖后的总面积不会超越 2^63 - 1 ，这意味着可以用一个 64 位有符号整数来保存面积结果。

解题思路

• 在二维坐标系中给出一些矩形，要求这些矩形合并之后的面积。由于矩形有重叠，所以需要考虑合并以后的面积。矩形的坐标值也会很大。

• 这一题给人的感觉很像第 218 题，求天际线的过程也是有楼挡楼，重叠的情况。不过那一题只用求天际线的拐点，所以我们可以对区间做“右边界减一”的处理，防止两个相邻区间因为共点，而导致结果错误。但是这一题如果还是用相同的做法，就会出错，因为“右边界减一”以后，面积会少一部分，最终得到的结果也是偏小的。所以这一题要将线段树改造一下。

• 思路是先讲 Y 轴上的坐标离线化，转换成线段树。将矩形的 2 条边变成扫描线，左边是入边，右边是出边。

  

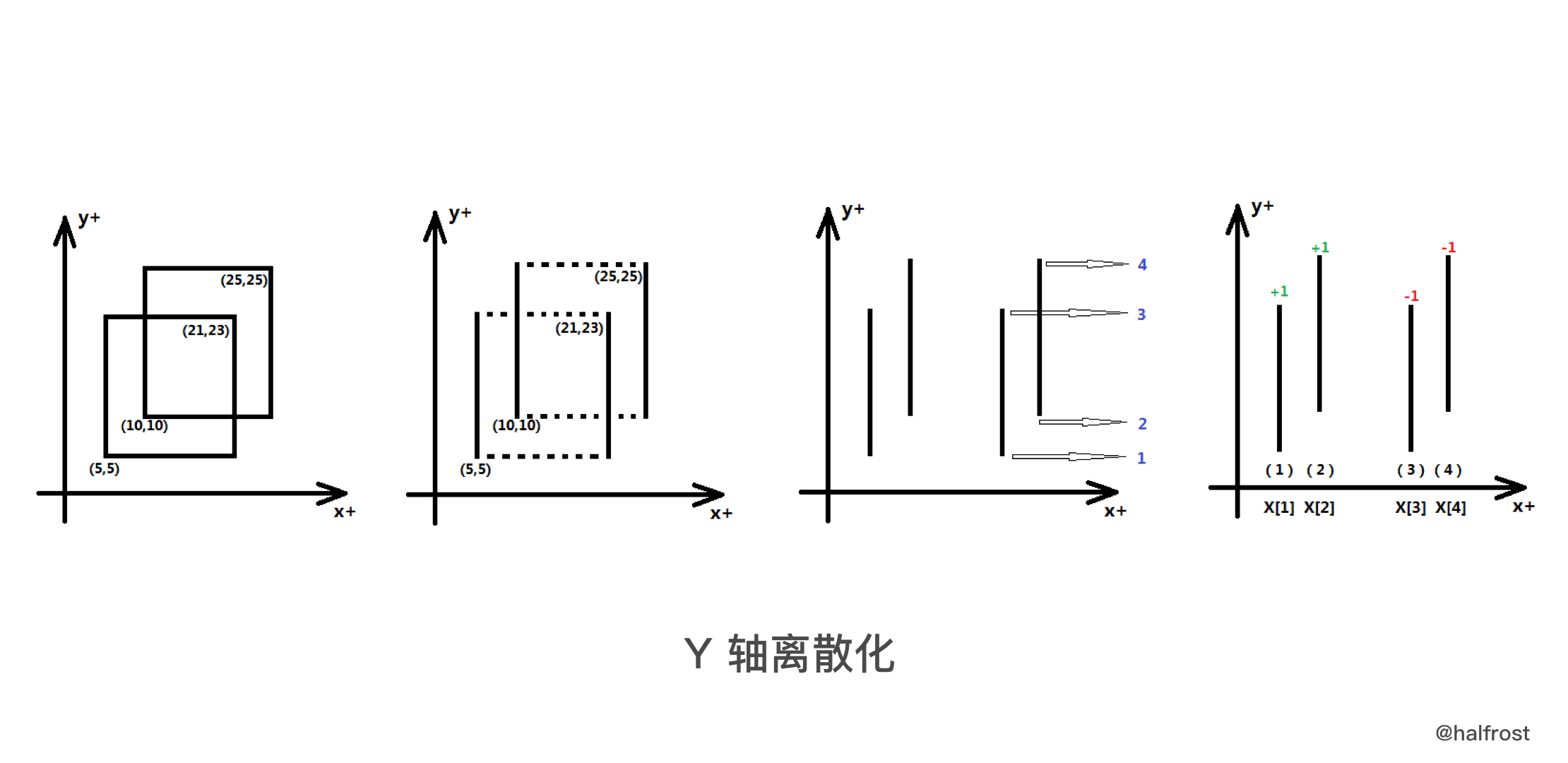
• 再从左往右遍历每条扫描线，并对 Y 轴上的线段树进行 update。X 轴上的每个坐标区间 * query 线段树总高度的结果 = 区间面积。最后将 X 轴对应的每个区间面积加起来，就是最终矩形合并以后的面积。如下图中间的图。

  

  需要注意的一点是，每次 query 的结果并不一定是连续线段。如上图最右边的图，中间有一段是可能出现镂空的。这种情况看似复杂，其实很简单，因为每段线段树的线段代表的权值高度是不同的，每次 query 最大高度得到的结果已经考虑了中间可能有镂空的情况了。

• 具体做法，先把各个矩形在 Y 轴方向上离散化，这里的线段树叶子节点不再是一个点了，而是一个区间长度为 1 的区间段。

  

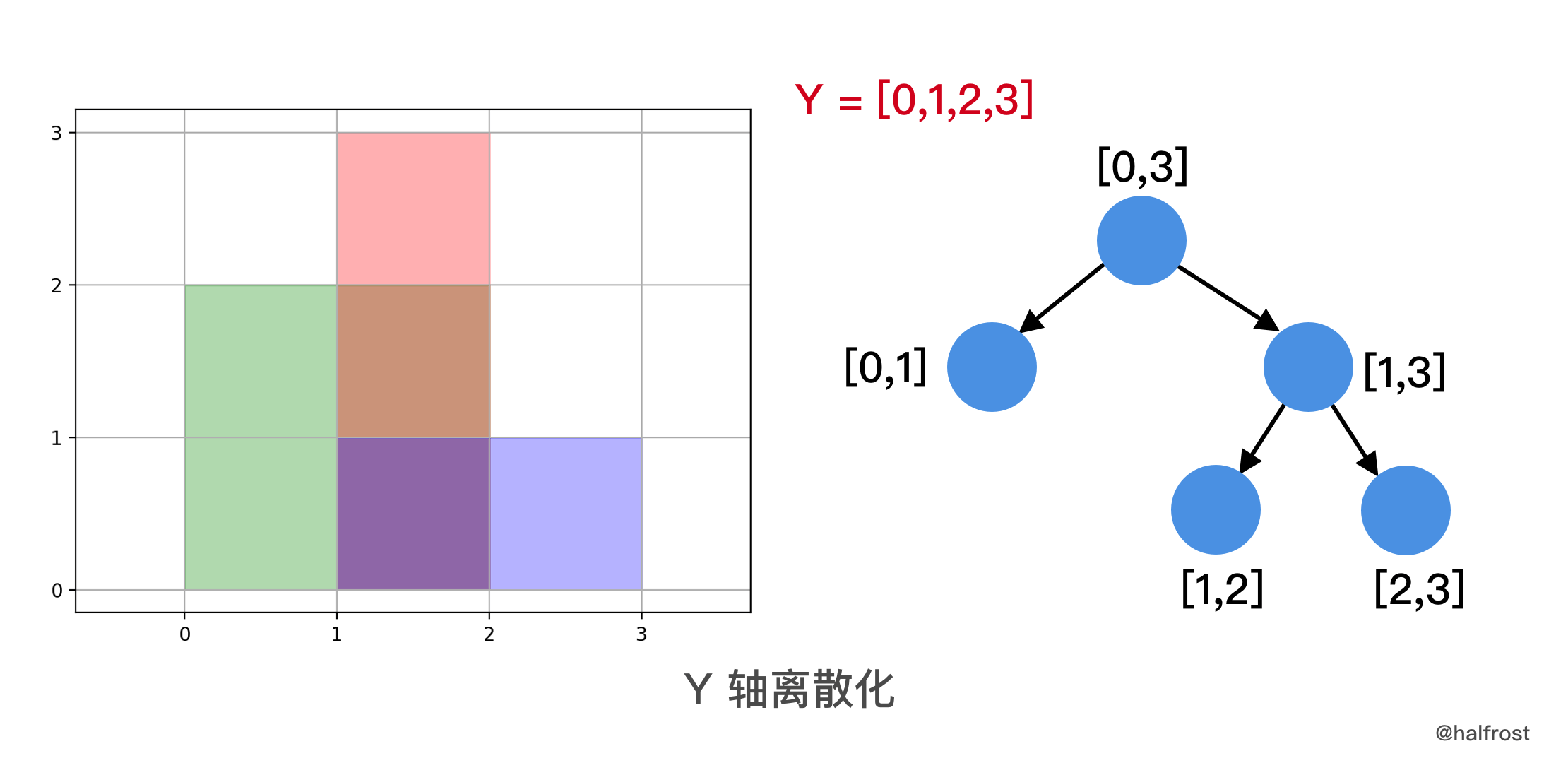
  每个叶子节点也不再是存储一个 int 值，而是存 2 个值，一个是 count 值，用来记录这条区间被覆盖的次数，另一个值是 val 值，用来反映射该线段长度是多少，因为 Y 轴被离散化了，区间坐标间隔都是 1，但是实际 Y 轴的高度并不是 1 ，所以用 val 来反映射原来的高度。

• 初始化线段树，叶子节点的 count = 0，val 根据题目给的 Y 坐标进行计算。

  

• 从左往右遍历每个扫描线。每条扫面线都把对应 update 更新到叶子节点。pushUp 的时候需要合并每个区间段的高度 val 值。如果有区间没有被覆盖，那么这个区间高度 val 为 0，这也就处理了可能“中间镂空”的情况。

  

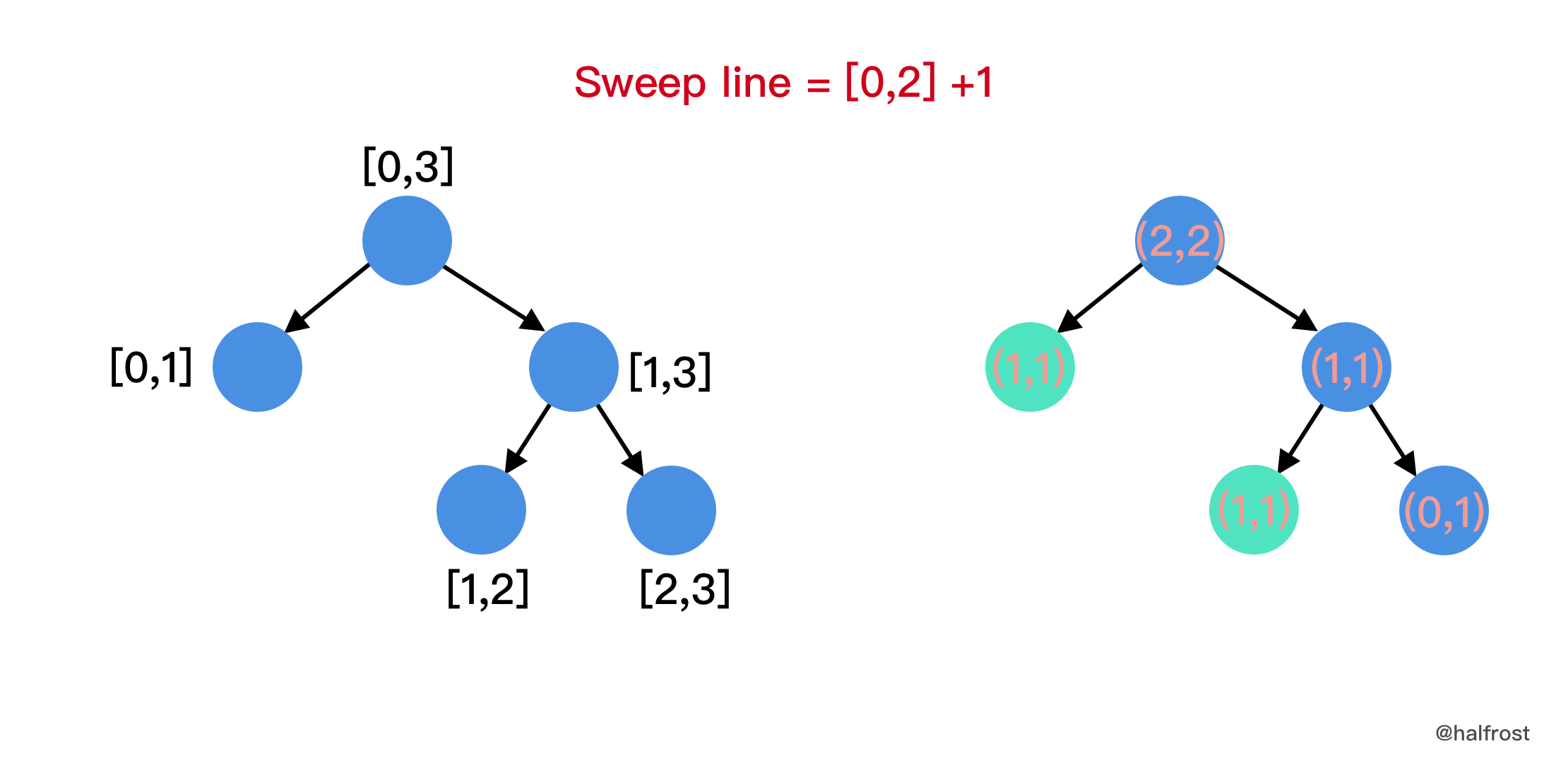
    func (sat *SegmentAreaTree) pushUp(treeIndex, leftTreeIndex, rightTreeIndex int) {
    	newCount, newValue := sat.merge(sat.tree[leftTreeIndex].count, sat.tree[rightTreeIndex].count), 0
    	if sat.tree[leftTreeIndex].count > 0 && sat.tree[rightTreeIndex].count > 0 {
    		newValue = sat.merge(sat.tree[leftTreeIndex].val, sat.tree[rightTreeIndex].val)
    	} else if sat.tree[leftTreeIndex].count > 0 && sat.tree[rightTreeIndex].count == 0 {
    		newValue = sat.tree[leftTreeIndex].val
    	} else if sat.tree[leftTreeIndex].count == 0 && sat.tree[rightTreeIndex].count > 0 {
    		newValue = sat.tree[rightTreeIndex].val
    	}
    	sat.tree[treeIndex] = SegmentItem{count: newCount, val: newValue}
    }
  

• 扫描每一个扫描线，先 pushDown 到叶子节点，再 pushUp 到根节点。

  

  

  

  

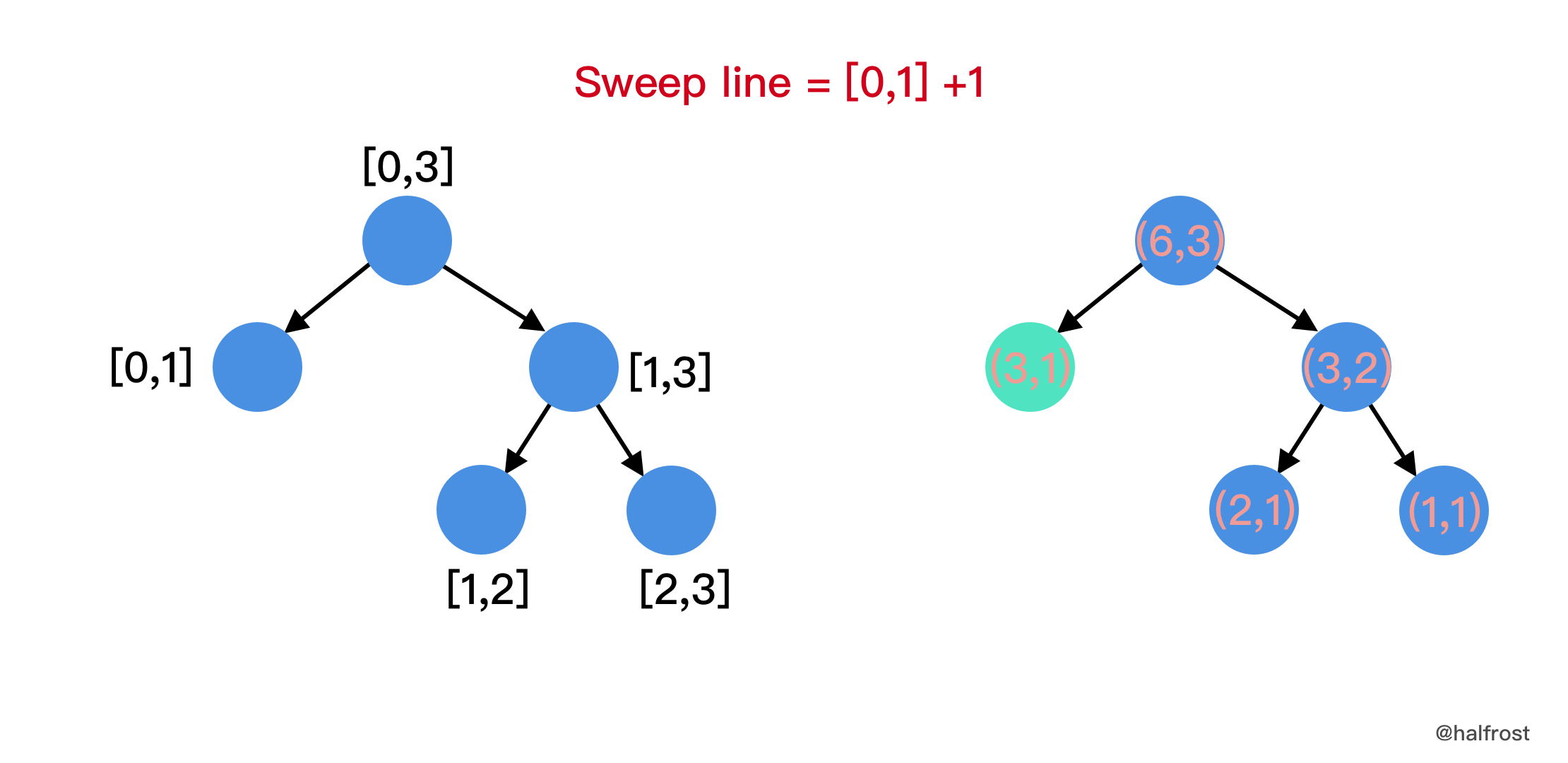
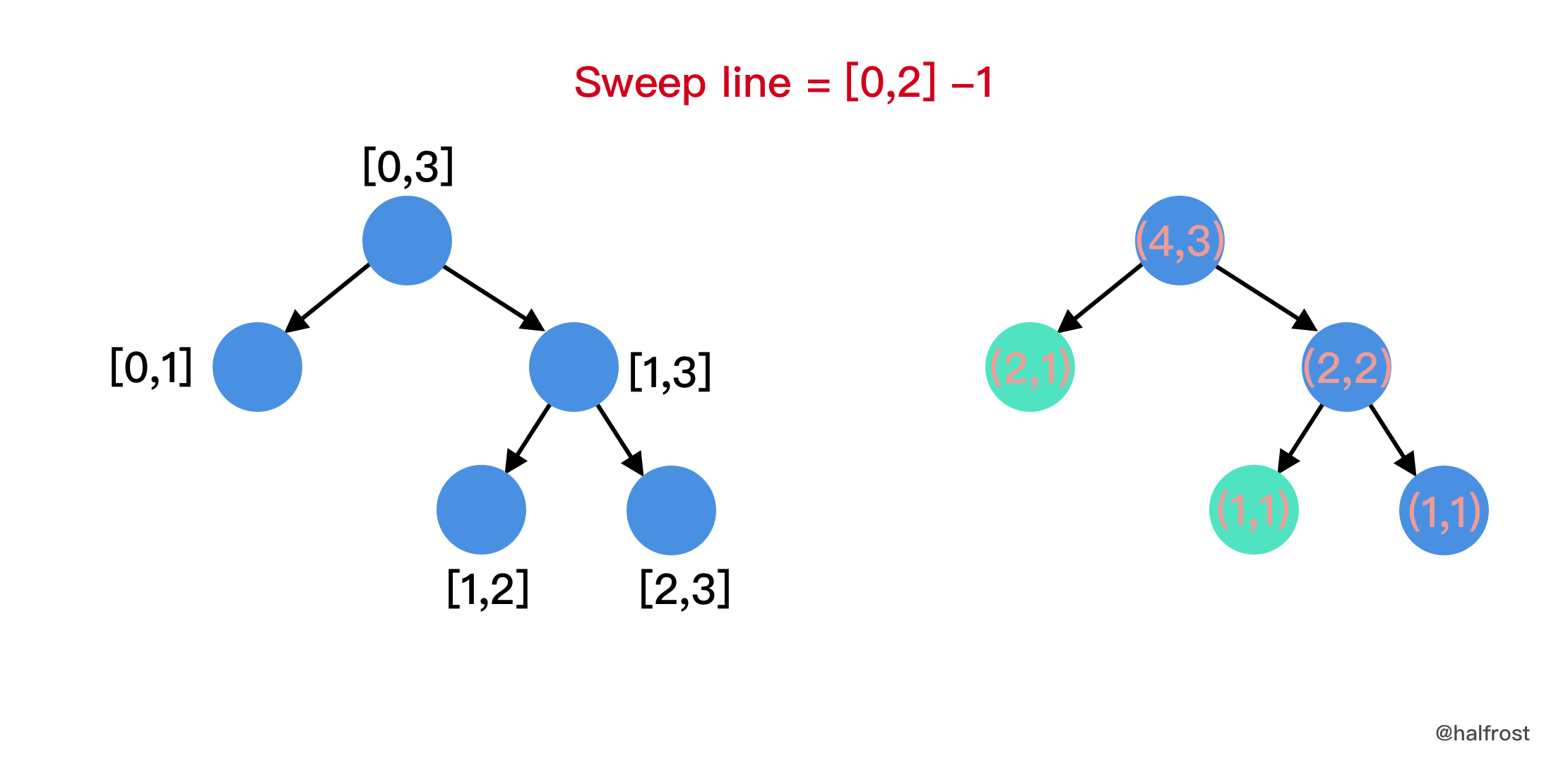
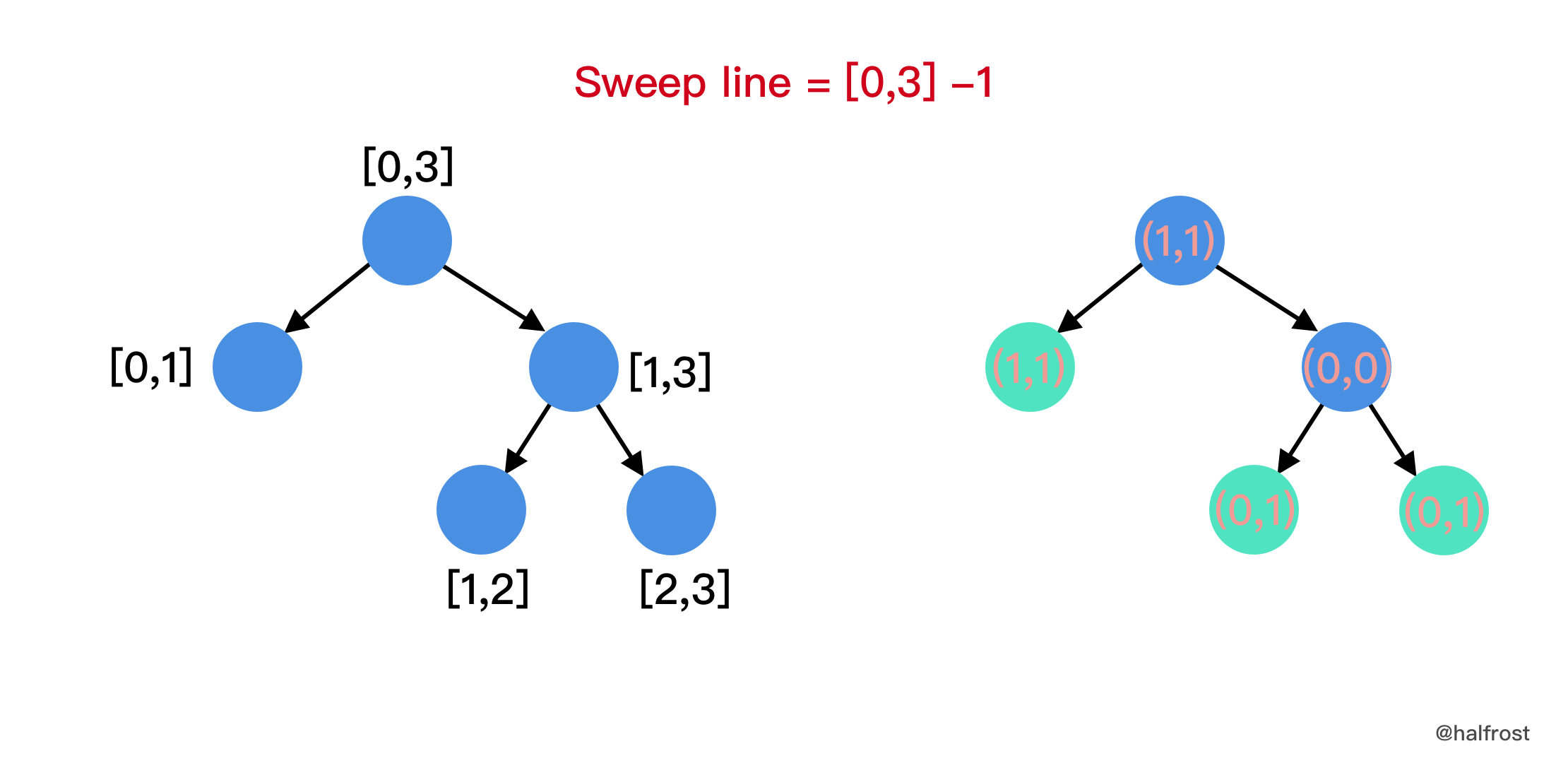
• 遍历到倒数第 2 根扫描线的时候就能得到结果了。因为最后一根扫描线 update 以后，整个线段树全部都归为初始化状态了。

  

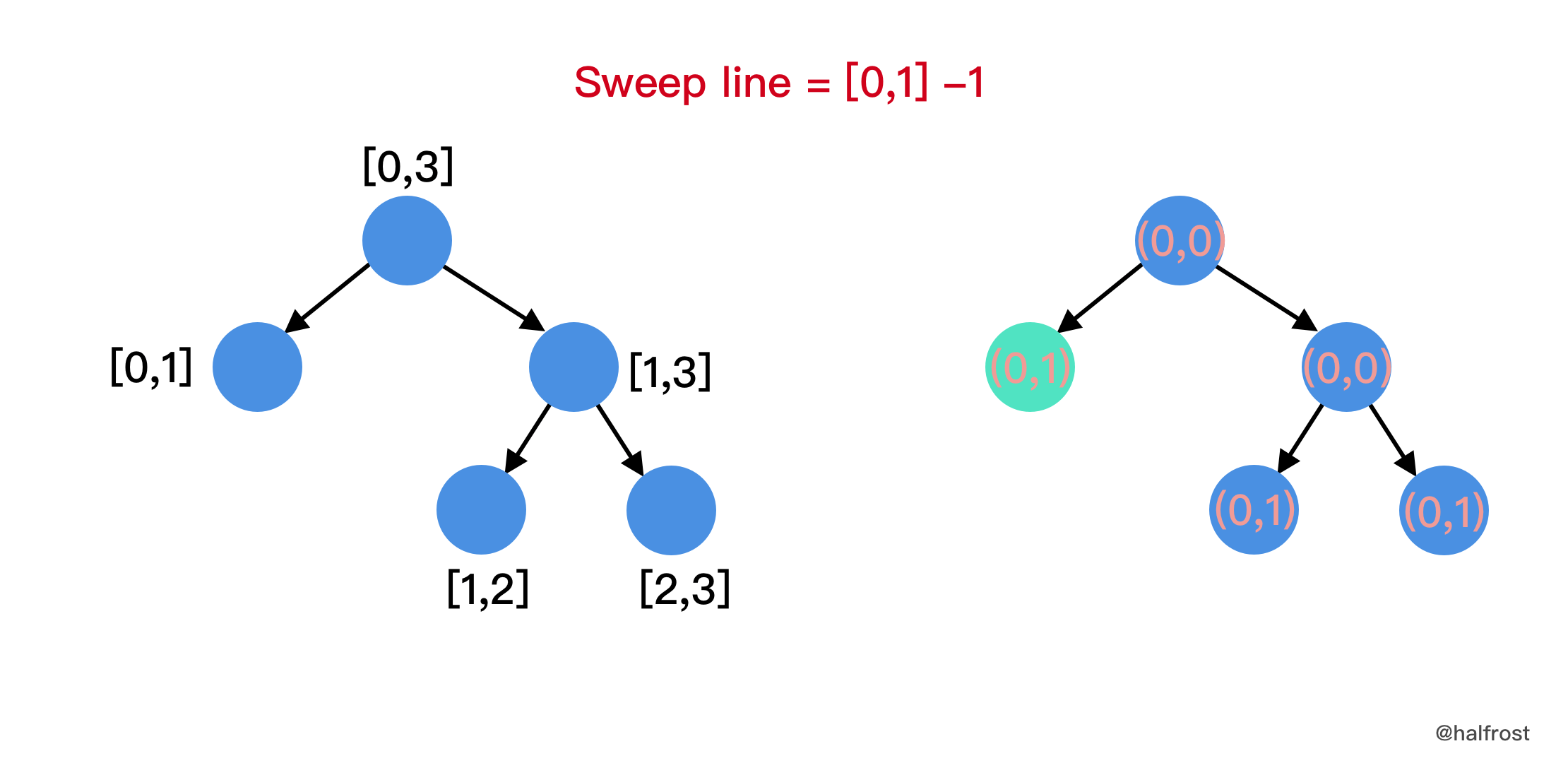
• 这一题是线段树扫面线解法的经典题。

Documentation

Index

• type LineItem
• type SegmentAreaTree
  • func (sat *SegmentAreaTree) Init(nums []int, oper func(i, j int) int)
  • func (sat *SegmentAreaTree) Query(left, right int) int
  • func (sat *SegmentAreaTree) Update(updateLeft, updateRight, val int)
• type SegmentItem

Types

type LineItem

type LineItem struct {
	// contains filtered or unexported fields
}

LineItem define

type SegmentAreaTree

type SegmentAreaTree struct {
	// contains filtered or unexported fields
}

SegmentAreaTree define

func (*SegmentAreaTree) Init

func (sat *SegmentAreaTree) Init(nums []int, oper func(i, j int) int)

Init define

func (*SegmentAreaTree) Query

func (sat *SegmentAreaTree) Query(left, right int) int

Query define

func (*SegmentAreaTree) Update

func (sat *SegmentAreaTree) Update(updateLeft, updateRight, val int)

Update define

type SegmentItem

type SegmentItem struct {
	// contains filtered or unexported fields
}

SegmentItem define